Amanda Stricklan, Tim Waters, James Klimchuk

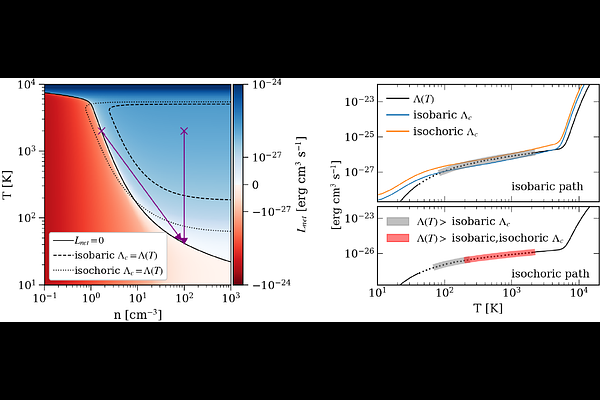
To model the temperature evolution of optically thin astrophysical environments at MHD scales, radiative and collisional cooling rates are typically either pre-tabulated or fit into a functional form and then input into MHD codes as a radiative loss function. Thermal balance requires estimates of the analogous heating rates, which are harder to calculate, and due to uncertainties in the underlying dissipative heating processes, these rates are often simply parameterized. The resulting net cooling function defines an equilibrium curve that varies with density and temperature. Such cooling functions can make the gas prone to thermal instability (TI), which will cause departures from equilibrium. There has been no systematic study of thermally unstable parameter space for nonequilibrium states. Motivated by our recent finding that there is a related linear instability, catastrophic cooling instability, that can dominate over TI, here we carry out such a study. We show that Balbus' instability criteria for TI can be used to define a critical cooling rate, $\Lambda_c$, that permits a nonequilibrium analysis of cooling functions through the mapping of TI zones. We furthermore extend Balbus' criteria to account for thermal conduction. Upon applying a $\Lambda_c$-based stability analysis to coronal loop simulations, we find that loops undergoing periodic episodes of coronal rain formation are linearly unstable to catastrophic cooling instability, while TI is stabilized by thermal conduction.