Nikyta Shchutskyi, Matthieu Schaller, Orestis A. Karapiperis, Federico A. Stasyszyn, Axel Brandenburg

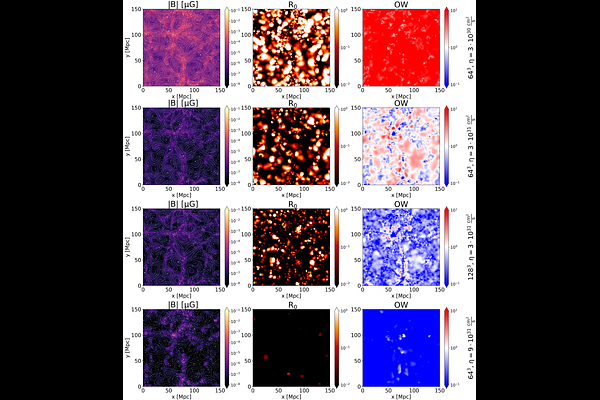
Understanding the origin and evolution of magnetic fields on cosmological scales opens up a window into the physics of the early Universe. Numerical simulations of such fields require a careful treatment to faithfully solve the equations of magnetohydrodynamics (MHD) without introducing numerical artefacts. In this paper, we study the growth of the magnetic fields in controlled kinematic dynamo setups using both smoothed particle hydrodynamics implementations in the SWIFT code. We assess the quality of the reconstructed solution in the Roberts flow case against the reference implementation in the Pencil code and find generally a good agreement. Similarly, we reproduce the known features of the more complex ABC flow. Using a simple induction-diffusion balance model to analyse the results, we construct an "overwinding" trigger metric to locally detect regions where the magnetic diffusion cannot counteract the expected induction because of limitations in the method's ability to resolve magnetic field gradients. This metric is then used to identify the necessary resolution and resistivity levels to counteract the overwinding problem. We finally apply this metric to adiabatic cosmological simulations and discuss the resolution requirements needed to resolve the growth of the primordial fields without artefacts.